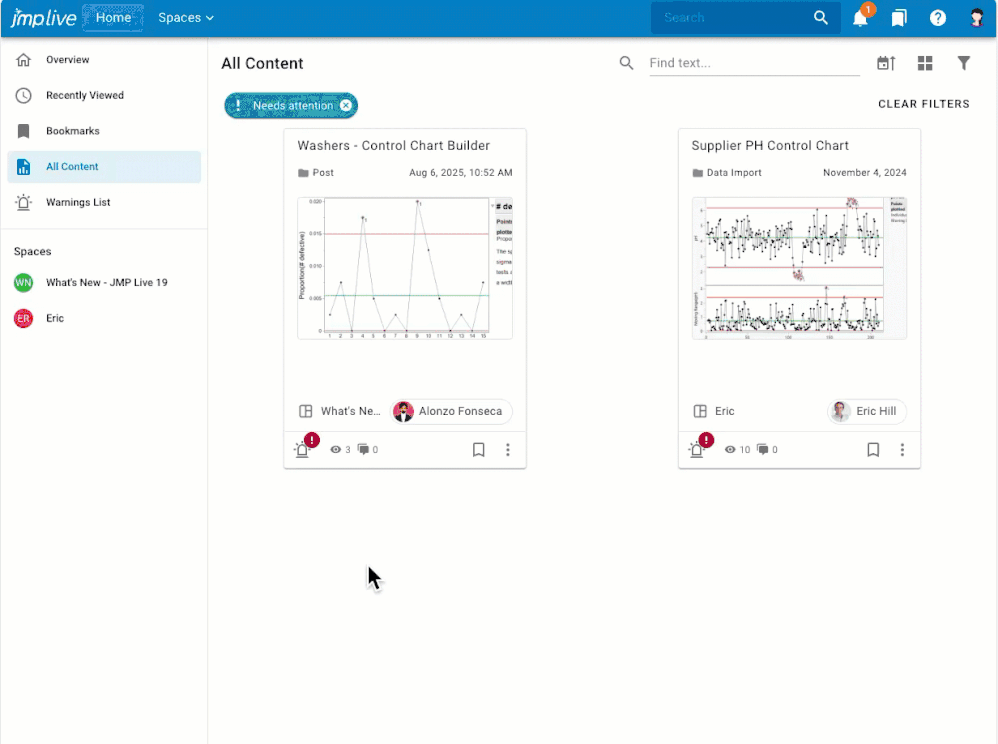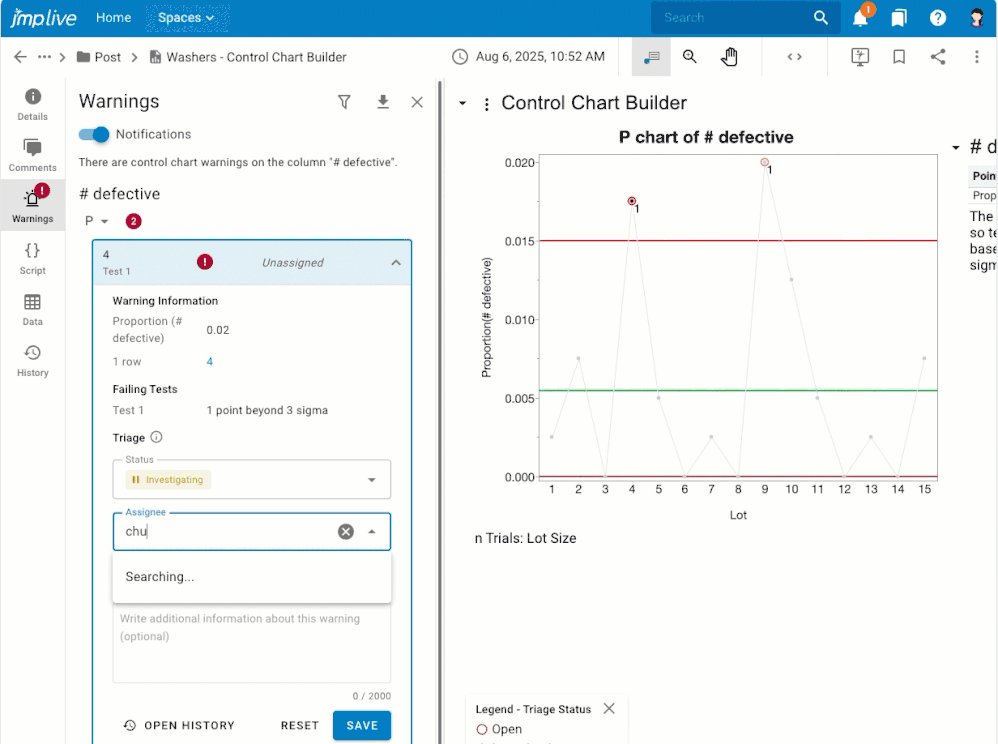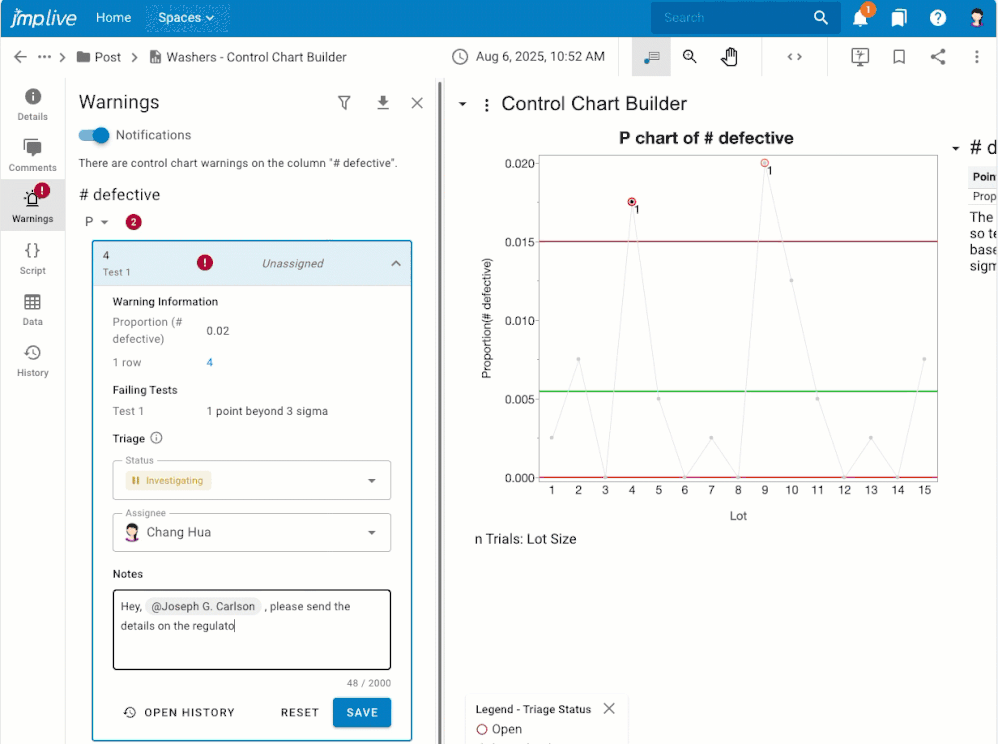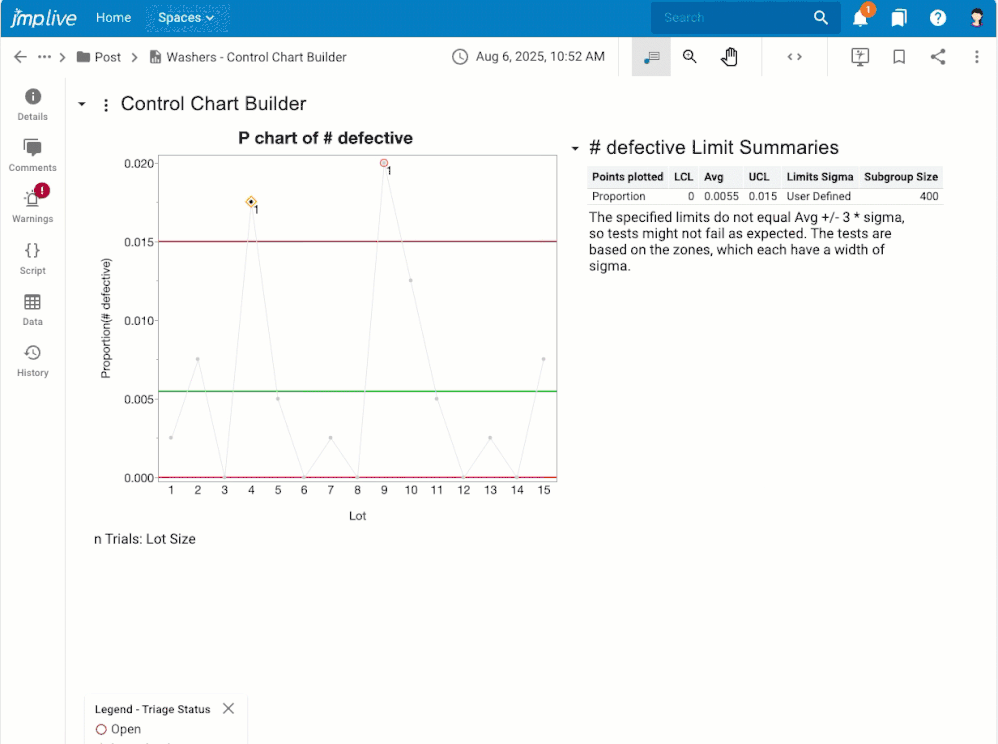
Unlock dynamic data stories: Tips for publishing on JMP Public
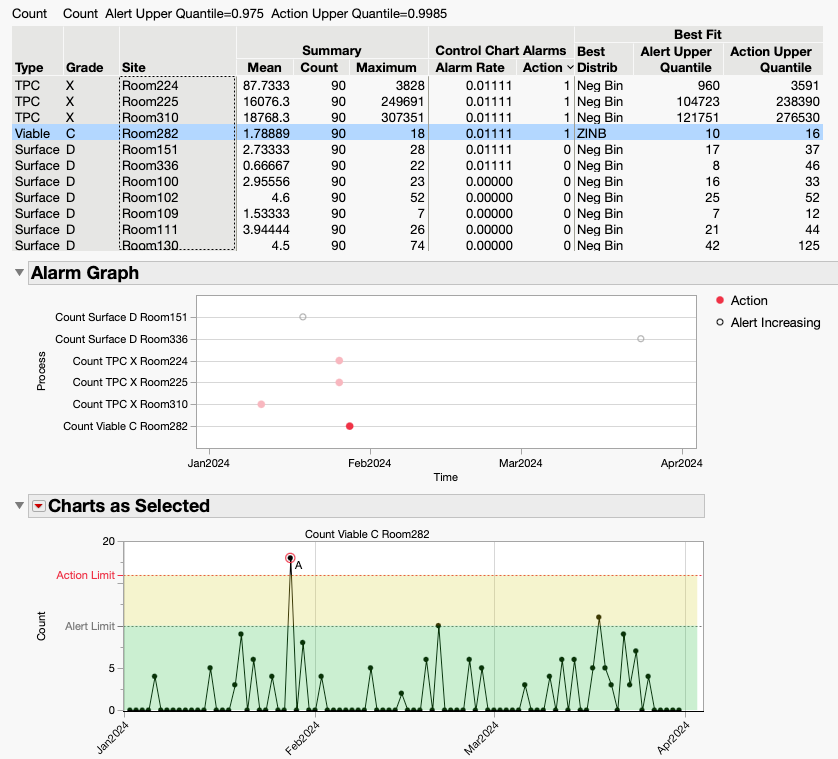
If you’re looking to make your data stories and presentations shine, forget static images and boring slides. JMP Public is a high-impact platform that showcases the dynamic, interactive power of JMP visualizations. Here are a few best practices to follow when posting: Enhance post interactivity and engagement If possible, share a report that will be interactive in JMP Public, such as a visuali...
 dieter_pisot
dieter_pisot

 josh_markwordt
josh_markwordt
 Hamid_Sanei
Hamid_Sanei
 Masukawa_Nao
Masukawa_Nao
 Craige_Hales
Craige_Hales

 anne_milley
anne_milley

 kristen-slappey
kristen-slappey
 MeichenDong
MeichenDong
 willmorgan
willmorgan
 geoffrey_mann
geoffrey_mann XanGregg
XanGregg

 julian
julian

 Aurora_TiffanyD
Aurora_TiffanyD