Hi all,
I just have a question when using fit Y by X function in JMP.
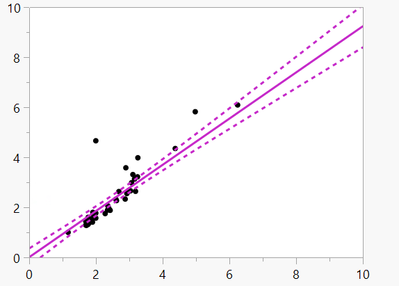
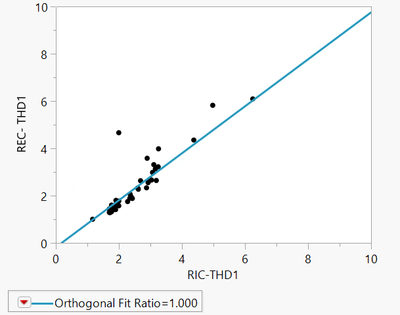
I use 2 method is linear regression & orthogonal regression for a data.
I know the difference is Linear method assume that there is only variations at Y value when Orthogonal assume variation of both X,Y.
But I wonder why can't I draw the Confidence interval or prediction interval in the orthogonal method.
I just can see the Lower CL & Upper CL & cannot draw the line.
is this Lower & upper CL is confident interval?
If yes can I somehow draw the CI into the chart?
Can somebody help to explain? Thank you very much