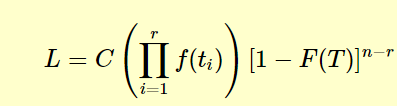- JMP User Community
- :
- Discussions
- :
- Re: Does JMP's loglikelihood value include a constant?
- JMP 19 is here! See the new features at jmp.com/new.
- Register to attend Discovery Summit 2025 Online: Early Users Edition, Sept. 24-25.
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Does JMP's loglikelihood value include a constant?
When JMP shows the loglikelihood value, does it set the constant to 0 or some number? Thanks!
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
In this context, the constant C is not a known quantity before seeing the data. In general, when one says the loglikelihood does not include a constant, that usually means that MLE is not affected by subtracting the constant from the loglikelihood. The constant can be anything that does not involve parameters. E.g. constant can be a function of data, a function of sample size, etc.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
It depends on where you see the loglikelihood. For Normal distribution related loglikelihood, the constant is a function of pi and sample size; for Lognormal distribution related loglikelihood, the constant adds a function of data as well. Including constant will increase complexity and time consumption in calculation. So the strategy is NOT to include the constant, unless we have to. E.g. in Life Distribution, constants are included, because there is a need to compare different distributions like between Normal and Lognormal, where the constants matters.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
For the single Weibull fitting and competing two Weibull risk mixture, what would the constants be? Thanks!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
Seems you are talking about loglikelihood in Life Distribution. Then the answer is about another "depends". If the data have failure observations, then the constant is the function of those failure time, just like the constant of a Lognormal distribution. If there are not failure observations, then there are no separable constant. For mixture, either regular mixture or competing risk mixture, I don't believe there are separable constants, at least in general. In Life Distribution, the loglikelihood is "full", so it does not deliberately differentiate a constant part and a non-constant part.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
For censored data, the likelihood function is:
So isn't the constant set beforehand, and not a function of data? Thanks!
Ref: https://www.itl.nist.gov/div898/handbook/apr/section4/apr412.htm
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
C can be different things for different distributions, depending on what is f.
For example, when fitting a Weibull likelihood, if f and F are density and distribution functions of Weibull, then there is not separable constant at all.
However, the likelihood is equivalent to a form, in which there is a constant C, and f and F are density and distribution functions of Smallest Extreme Value (SEV) distribution. I guess that is what the NIST formula is depicting.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
Since JMP is giving the loglikelihood value, then when taking the log form of the formula, doesn't the C constant become separate? That's why I'm confused, because I thought that the constant could be set this way.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Does JMP's loglikelihood value include a constant?
In this context, the constant C is not a known quantity before seeing the data. In general, when one says the loglikelihood does not include a constant, that usually means that MLE is not affected by subtracting the constant from the loglikelihood. The constant can be anything that does not involve parameters. E.g. constant can be a function of data, a function of sample size, etc.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us