How to optimize factors with uncertainty in 1 step with JMP?

[#1]

Hello community!!,

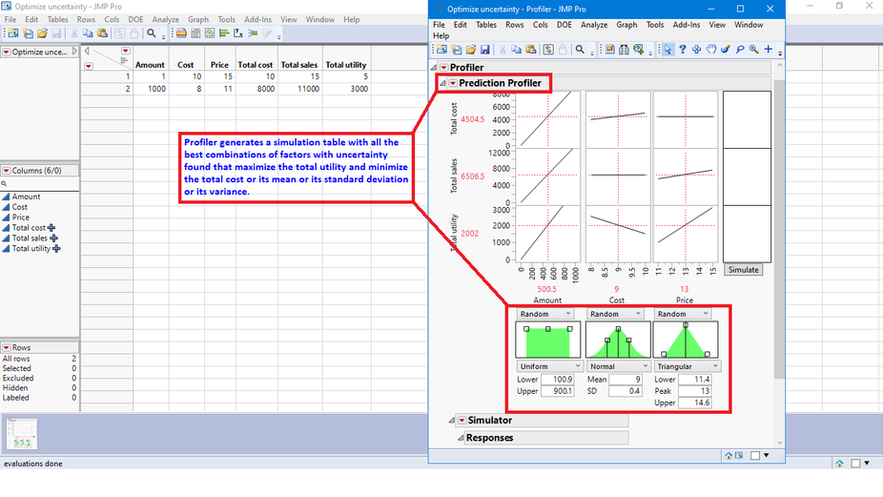
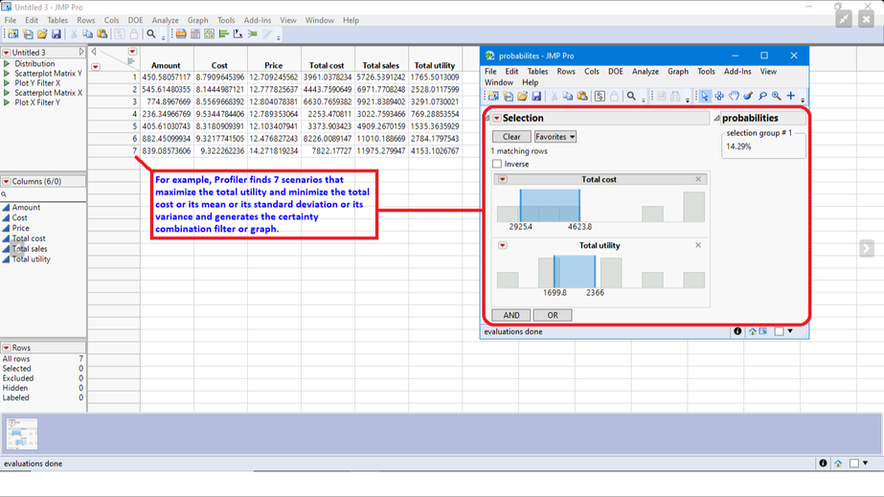
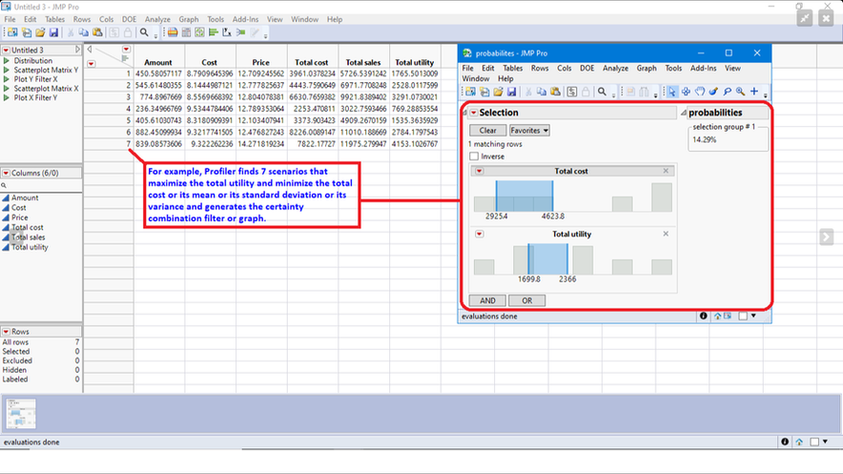
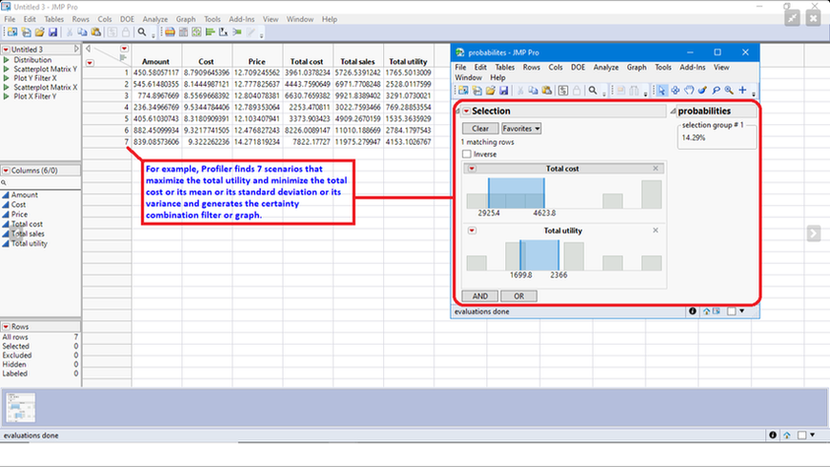
A question: will it be possible for the profiler to find the best combinations of factors with uncertainty when performing a simulation, which maximize the total utility and minimize the total cost, generating a table of the best combinations found? For example:

[#2]

Hello Mark,

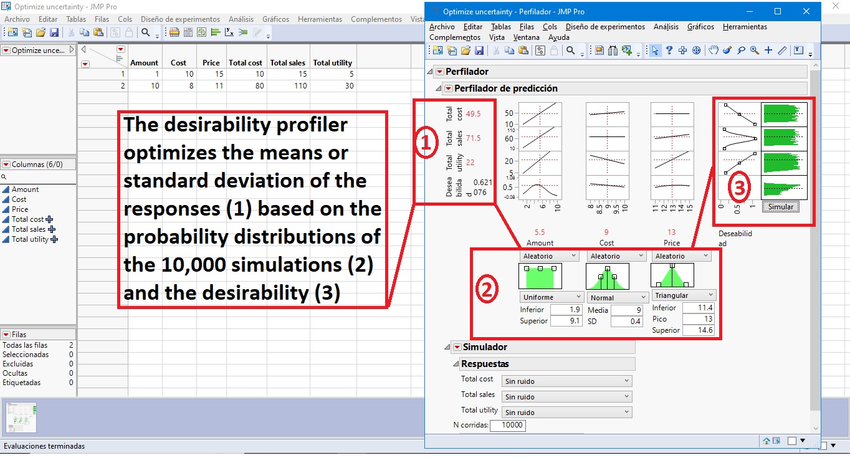
Thank you for your response, a question...will there be a way for JMP to optimize the mean or standard deviation of the responses of the total simulations generated?...that is, for the desirability profiler to use the probability and desirability distributions to find the combination of factors that achieve the desirability.

[#3]

The  by @yuichi_katsumur provides some additional possibilities to analyze the uncertainty of a prediction model, mainly with the goal to find spots with large potential information gain (~ uncertainty) - to optimize the model by adding such points for further experiments.

I guess one can use the same formulas, change the algorithm and use it for your task as well?

[#4]

The Bayesian optimization plugin is an interesting alternative for 1 objective and it would be better if it could optimize multiple objectives.
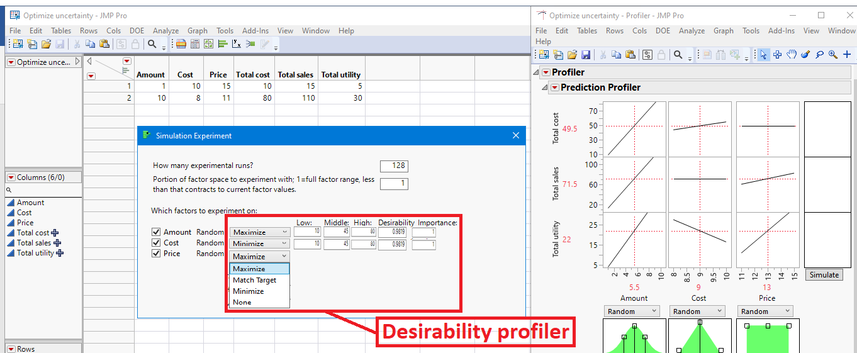
In relation to the simulation experiment, the desirability profiler should be included as an option so that in a single step it optimizes the mean responses or standard deviations or values and generates a table with the best responses found.

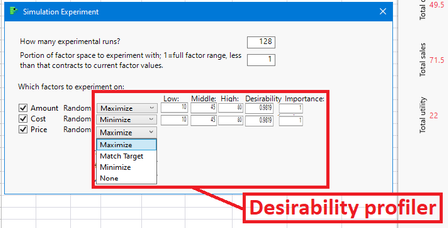
The idea I'm referring to is a plugin something like this:

[#5]

And generate a table with the best 1 or 2 or 3 or xxx results found of the mean or standard deviation or the value, for example:

[#6]

How to link these 2 kinds of simulations?

1) you have a model which you trust -  and you have some variations of the input parameters. Then one can find the optimal setting of the input parameters to optimize the outcome - specified by desirability. This approach neglects any uncertainties of the model.

2) with a specific setting of the input parameters, one performs experiments. But the model is not 100% nailed down: You invest at the stock market, you have some model about the possible outcome, but the outcome is not 100% clear yet. This leads to a certain spread of the possible resulting values - an uncertainty.

The second approach can be simulated by adding some additional parameters with variation. The variation of the factor settings is irrelevant for the optimization, but the variation of such additional factors isn't. Is it possible to keep such input factors fluctuating for the Optimization step, to weight the (un)certainty of the predicted value as part of the desirability?

---

@Mark_Bailey wrote:

With the desirability defined, click the red triangle next to Prediction Profiler and select Optimization and Desirability > Maximize Desirability. The variation in the factor settings is irrelevant to the search for the optimum factor settings.

---

[#7]

Hello Hogi,

I couldn't understand your answer (Google Translate), a question....
Will there be a JMP script or plugin that incorporates the desirability profiler into the simulation experiment platform?

And generate a table with the best 1 or 2 or 3 or xxx results found of the mean or standard deviation or the value, for example: