- JMP User Community
- :
- Discussions
- :
- How to find best fit values for a and b in following function y=1-exp((-x/a)^b) ...
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
I have data, I'd like to find the values of a and b in the following function that best fits the data: y=1-exp((-x/a)^b).
I'd also like to fit y=1-exp(-x/a) to see which function has the better fit.
Is there a way to do this? I'm using JMP 14.
Relaxation function vs hours annealed in attached file.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
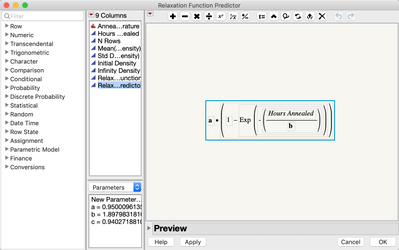
@Mark_Bailey is right. The Nonlinear platform is flexible and can fit any model you can parameterize in the Formula Editor.
Fit Curve and Nonlinear can work together since Fit Curve can save a parametric prediction formula.
So, after using Fit Curve to fit your Weibull Growth model you can save the parametric prediction formula. Use the Formula Editor to edit the formula and remove the exponent parameter (set set it to 1).
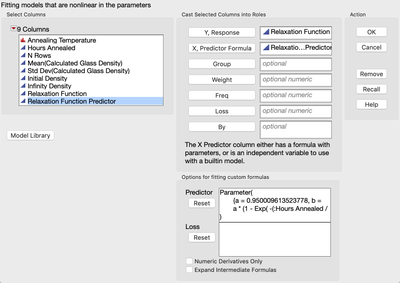
Then use the Nonlinear platform with your original column in the Y role and the new predictor function column in the X role.
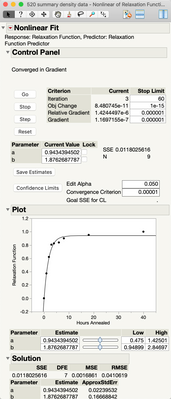
Click the Go button at the top of the report and you'll get estimates for the a and b parameters.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
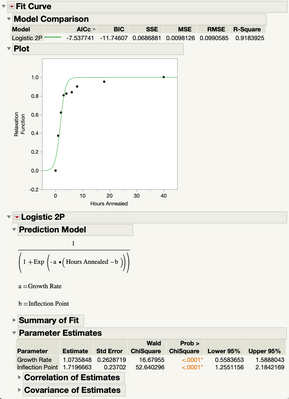
Take a look at the Fit Curve platform and the Logistic 2P curve. I think that's what you're looking for.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
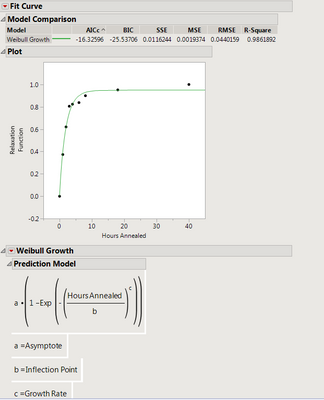
Thanks, I checked out fit curve and it looks like Weibull Growth is actually closest to what I want, but not exactly. Is there a way to force a variable, say a, to equal 1 so the equation matches what I'm looking for. Then the second equation I want to fit would have a and c equal 1.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
There are two platforms for non-linear models. Fit Curve is newer. It is based on a library of such models. If your model is one of them, then it is probably the best platform. The Nonlinear platform, though, has the advantage here because you can specify a custom model as a column formula. You can also manipulate the parameters during and between fits.
See JMP 14: Help > Books > Predictive and Specialized Models > Nonlinear chapter. There are lots of examples, too.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: How to find best fit values for a and b in following function y=1-exp((-x/a)^b) to fit data points
@Mark_Bailey is right. The Nonlinear platform is flexible and can fit any model you can parameterize in the Formula Editor.
Fit Curve and Nonlinear can work together since Fit Curve can save a parametric prediction formula.
So, after using Fit Curve to fit your Weibull Growth model you can save the parametric prediction formula. Use the Formula Editor to edit the formula and remove the exponent parameter (set set it to 1).
Then use the Nonlinear platform with your original column in the Y role and the new predictor function column in the X role.
Click the Go button at the top of the report and you'll get estimates for the a and b parameters.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us