- JMP User Community
- :
- Discussions
- :
- HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
How do I carry out a fractional factorial design of experiment. I have watched some videos but they did not explain the basics to me very well. I have a data I need to analyse but I am new to design of experiment, yet, I don't have time to spare. I will prefer an interactive explanation.
I greatly appreciate any help provided
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
I suggest you go through the DOE tutorial that is downloaded onto your computer when JMP is installed. It will walk you through the implementation of a Fractional Factorial Design
It can be found at:
Help==>Tutorials==>DOE Tutorial
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
The JMP tutorials are quite good for beginners. If you attach your data set to this thread, I'm sure someone will take a look and help you analyze it.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
Attached is my dataset. the last variable is the outcome variable (Y). All the others go into the model. Thank you very much
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
You will first need to tell us what change in the response variable (Y, Dried Cell Weight) is of practical value? What is the smallest increment of change that matters to you and what is the target value? Also did you keep track of run order?
Looking at your design, you have 4 treatments at the bottom with vastly different level setting? These are not center points. Curious as to how you came up with these treatment combinations?
First look (again this is a blind look as I don't know if the variation created has any real value):
1. You have an unusually large result for the treatment where the result is 0.706. This large value (special cause like) is likely due to noise in the experiment not due to treatment effects so be cautious in using quantitative analysis. This potential inflates experimental error.
2. I did the analysis without the last 4 treatments and attached the analysis (JMP Journal) using a saturated model. This is a screening of the linear effects of each of the factors and associated interactions (you have started with a rather high resolution design). Typically, you would simplify the model by removing insignificant terms and re-run the analysis to get residuals to assess the adequacy of the model. To do this, first you must determine if these results are of any practical significance.
3. I played with removing terms that met a practical significance of a .05 change in Y (Pareto Plot) and also showed statistical significance (Half normal plot). The results of this analysis are also in the attached journal. The residuals look a bit bimodal and there may be an outlier as well indicating there may be some issues with the model. I saved the residuals and the prediction formula for this analysis.
4. I then added the additional 4 treatments you had in your original data set. The prediction formula did a very poor job of predicting those 4 points (perhaps not unusual since those 4 points were well beyond the design space inference.). Looking at factor B, this factor appears to have a non-linear effect.
I'm sure others may take a different approach...let me know if you have any questions.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: HELP WITH FRACTIONAL FACTORIAL DESIGN OF EXPERIMENT
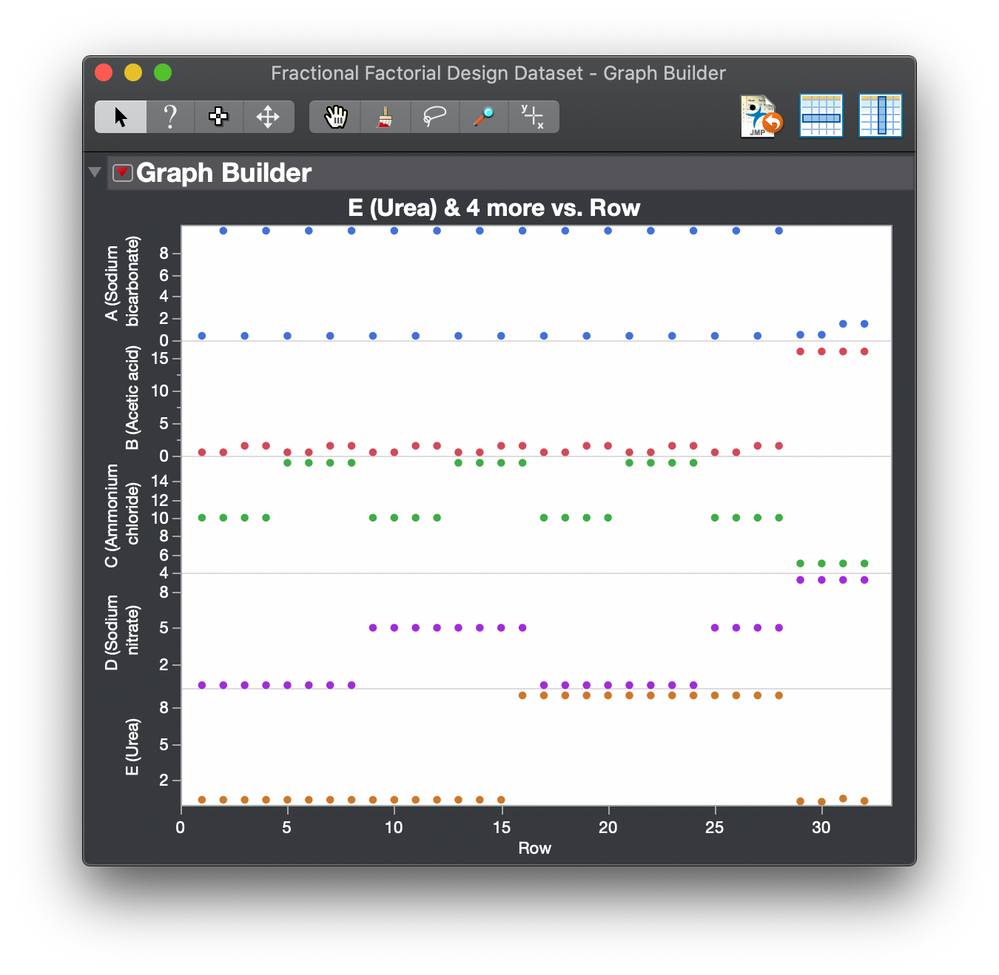
Adding to @txnelson and @statman , I plotted factor levels over the runs assuming that the order in the table is the order of the runs. I see a distinct pattern that suggests this experiment was not randomized.
The restriction on the randomization can dramatically affect the analysis. Regression of the linear model with a single error term (residuals) will inflate the type I errors of the hard to change factors and inflate the type II errors of the easy to change factors. It looks like you have five levels of randomization before the last four ad hoc treatments.
I also added column properties that facilitate the regression analysis of data from an experiment. JMP automatically adds these properties when you use JMP to design the experiment and make the table. You imported this data from an Excel workbook, which has no such properties.
I used Generalized Regression with Ridge regression to shrink the estimates and LASSO to select the parameters for a linear model with main effects and interaction effects, but if this experiment was not randomized, then I doubt any regression is valid.
- © 2024 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us