- JMP User Community
- :
- Discussions
- :
- Re: Capability analysis
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Capability analysis
Hi have continuous data (processing time)
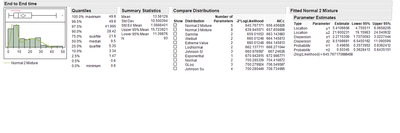
The Continuous Fit suggests the "Normal 2 Mixture Distribution"
Can I make a capability analysis with this distribution?
Thanks
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
bump
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
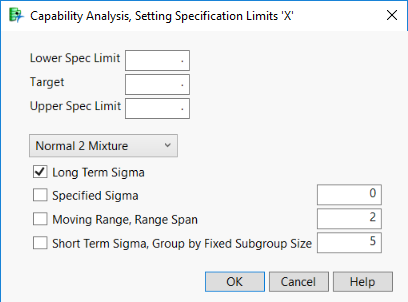
Yes, easily. In the capability analysis dialog within Distribution, you'll be able to choose a distribution for the data. Normal 2 Mixture is an option.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
Cameron
Are you using JMP pro, or maybe it is a new feature in JMP 14? I'm using JMP13.2.1 standard and I don't think I have that feature.
My two cents on the topic is that if I care enough about a given parameter, I would make sure I understand why the distribution is multi modal, understand how close the modes are to one another, whether they're stable, and decide whether it needs to be or can be "fixed." If not then I'd apply some robust stats to it. For example either JMP's robust mean / standard deviation to get a robust cpk, or use a function of median and iqr to do the same.
-Mike
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
I'm using base JMP version 13.2. You can also get the capability analysis for any of those fitted distributions by clicking the red-arrow icon for the fit and selecting "Spec Limits". Setting those will generate a capability analysis.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
If the normal mixture model is a valid description of the data, then it implies that there is more than one normal population. What does it mean to ask if they are stable and capable as in the case of one population? That they are individually stable and capable? That the mixture of the two is stable and capable?
Why is there more than one population? Can they be identified?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
"Stable" may not have been the correct term, but as an example of unstable: There might be some problem with 1 piece of test equipment for used for one lot in the factory, where a subset of the data shows up as a new mode. I would not want to include this aberrant data if I was looking to set cpk based limits.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
"set cpk based limits?"
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Capability analysis
For some types of parameters, the distribution is such that it doesn't make sense to do this, usually cases of test measurement limitation or some idiosyncrasy of the product. Or if it is a resistance measurement, and the statistically set lower limit is negative, I adjust that to a positive value as electrical resistance cannot be negative. In these cases it is simple enough to eyeball some limits, though I generally try to apply robust limits first and if they don't make sense (too wide, too narrow, etc), I'll just manually implement a sensible limit for the parameter. Criticality of the parameter itself is taken into account.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us