- JMP User Community
- :
- Discussions
- :
- Re: Which Control Chart to use?
- Register to attend Discovery Summit 2025 Online: Early Users Edition, Sept. 24-25.
- New JMP features coming to desktops everywhere this September. Sign up to learn more at jmp.com/launch.
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Which Control Chart to use?
Just joined the community today and had a question about control charts. I am collecting data that consists of 120 measurements taken once a minute. My goal is to understand the variation between the measurements (between 1 and 2, 2 and 3, etc.) and I have the measurements in groups for each minute of time (120 measurements for 12:01, 120 measurements for 12:02, 120 measurements for 12:03, etc.). Based on what I am trying to understand and the way in which the data is collected, which control chart would be best to use? I tried searching a few discussions on this, but was still confused whether my data is continuous or is actually divided into sub groups.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
You'll want to insure process stability (or at least assume it?) by control charts before assessing process capability. Then check for normality of the individual observations...JMP can calculate process capability indices for select non-normal distributional forms so just make sure you check for the appropriate non-normal distribution if needed.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
Peter,
In order to use Process Behavior Charts such as the X-mR or I-mR charts for individuals and/or subgroups, normality of the data is NOT required nor assumed. This is one of the great myths regarding Control Charts. You can find this in Shewhart's original work (Shewhart invented the control chart) or in many articles written by Dr. Donald Wheeler. W. Edwards Deming had a lot to say on this subject as well.
That said, some Attributes control Charts DO require certain distributions: NP-charts for counts and P-charts for proportions require the binomial distribution. The C-chart for counts and U-chart for rates require the Poisson distribution.
to paraphrase Dr. Shewhart: "The normal distribution is neither a prerequisite for, nor a consequence of a process being in statistical control." I have confirmed this many times in my 30+ years of utilizing statistical tools.
"Normal distribution? I've never seen one." - W. Edwards Deming (May, 1991 4-day seminar in Cincinnatti, which I attended)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
smoore2: I am well aware of the issues you raise regarding normality and the use of control charts. My comments around normality were related to the original poster's questions around issues surrounding process capability analysis...where the shape of the distribution becomes very important.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
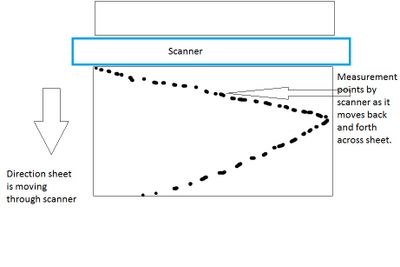
Do you have a scanner that is moving back and forth across the sheet as the sheet is moving through the equipment, or are you measuring 120 points across the sheet at the same time? If the former is the case, can you stop the scanner and test one place across the sheet multiple times? This kind of information is needed to be able to help you the best way I can.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
smoore,
Yes it is the first situation you are describing. The scanner is moving back and forth across the sheet as the sheet is moving through the scanner. I have recently found out that the raw data from the scanner is actually measuring 600 points and that the 120 points are an average from the 600. Also I cannot access the raw data, I am only able to examine the 120 points. Is there different criteria if I am dealing with average values instead of individual measurements? I have attached picture that shows what the sample points actually look like. This is a pretty crude drawing, but I just wanted to describe how the measurement points are "landing" on the sheet.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
Yes, this is exactly the same as scanners in the paper industry, which measure such properties as basis weight, moisture content, optical properties, etc. Paper is notorious for having a profile in the cross direction as well as a long-term profile in the machine direction. While this makes it more difficult to handle purely statistically, I have found that you can by-pass some of these issue to get at the underlying structure of the data and learn what the process is doing. Don't let "computation triumph over content", as Dr. Wheeler often says. I have found that the best way to perform studies with a scanner is to put the scanner in "single point mode" in different "lanes" across the web so that you can compare lanes (i.e., the profile). In paper, we make rolls of paper and at the end of each roll, the scanner data is summarized and broken down into cross-machine and machine-direction components. These summaries are often useful in and of themselves.
Again, please e-mail me and/or we can talk by phone if I can be of help. It is difficult to express everything that needs to be discussed in a forum such as this.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
Looking back, I think I have the cart before the horse. My original goal in trying to determine what control chart to use is really to see if the process is in control. The reason for this is that I would like to complete a process capability study to determine if our process can consistently meet customer specifications. Now, even through 120 measurements are taken, and it can be seen that there is variation between the measurements; these 120 measurements are averaged to a single value and this value is used to make adjustments. The process is not actually controlled based on the variation between the individual measurements across the sheet, but rather the average of the 120 measurements.However, to improve the process I need to reduce the variation between the measurements....would the following through process be correct:
1) First plot the Xbar-R chart to see if there are measurements from the sub groups which are out of the control limits (using data taken each minute over a half hour)
2) For those sub groups that are outside of the upper and lower control limits, use an IM-R charts to look for special cause variation.
Does this sound reasonable?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
If you aren't sure if your process is in control, you probably want to do a variability study first. This would allow you to look at all the sources of variability and see if the assumptions required for an effective implementation of SPC are being met. This study can be found under Analyze > Quality and Process > Variability / Attribute Gauge Chart. You can do a components of variance analysis among other useful analyses before you "throw the switch" on an SPC implementation.
Best,
M
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
Michael,
The only way to understand if your process is in control is to construct a control chart first!!! Your statement seems to follow the old myth that a process has to be in control before a control chart can be used. The real question: Is the process homogeneous? The only way to answer is to start with a properly constructed control chart. Almost any study in which tiime-ordered data is available should START with control chart(s) before doing anything else. I know this idea flies in the face of "Six Sigma", but the failings of "Sick Sigma" is another topic for another time. Please see the wotk of Donald Wheeler and Davis Balestracci to understand further what I am saying here. They both have published plenty of great information on the internet (esp., www.qualitydigest.com).
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Which Control Chart to use?
Dude! Why you gotta' hate on L6s? Lets keep it friendly in the community.
I also appear to have hit a nerve here... let me say that I like Wheeler's work. I have 4 of his texts and draw from them extensively. Since you mentioned L6S, I should also mention our trainer drew from his works extensively for our SPC training. But most of my thoughts on this issue predated my BB certification by a few years. I also think that my industry bias might have made it hard to understand my point.
My personal bias is that, while the world won't stop turning if I just dump my data into a run chart and calculate some limits, I absolutely need to check that my components of variance and variability charts support any decisions I make from the data. It doesn't cost anything to do this any more than just generating the run chart does. This is primarily because, for semiconductor manufacturing - particularly at the newer technology nodes, we can not make the assumption our measurement error is negligible relative to our signal of interest. So, doing the sanity check of gauge and MSA studies is industry best practice.
The gauge studies and CoV analyses (particularly the graphics that are part of the JMP platform) are also useful in determining where the point of homogeneity is and identifying correlations in the data. This second point is a very serious issue for semiconductor due to some of the physics in the manufacturing process. These points are quite important, as you and others on this thread have made abundantly clear, as some of the assumptions required for good SPC are invalid if the rational subgroup is not chosen correctly. SO, rather than saying invtanofmark should prefer one over the other, I propose that invtanofmark should never look at one without the other - that they are complementary. In my workflow I would do the gauge and CoV first to determine where my rational subgroup size is so that my control chart makes sense right out of the gate and I didn't have to go back and make adjustments - when you're managing several thousand metrology SPC charts every second saved counts!
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us