- JMP User Community
- :
- Discussions
- :
- Sample Size and Power (One Sample Proportion?)
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Sample Size and Power (One Sample Proportion?)
Dear Community,
I write you for a specific topic on sample size.
I know that JMP can support it… but… I need some details.
Question.
- I have the Process A;
- I process 100 samples and 10 of this failed the final test. This means: process Yield = 90% (or process scraps = 10%);
- I change some parameters on the process to eliminate the scrap. This means that I want: new process Yield = 100% (or process scraps = 0%);
- How many samples I need to process (with the new setup) to validate the 100% of yield (or 0% of scrap) with the new setting (with a specific level of confidence -> for example 95%);?
I think I need to use “Sample Size & Power” (One sample proportion)… but I’m not sure on this.
Thanks in advance for your feedback.
Best Regards,
Simone
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Sample Size and Power (One Sample Proportion?)
I think that this power calculation is correct for your case. Please see the documentation for help with setting up the power analysis.
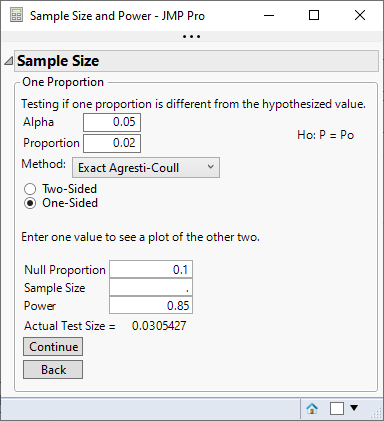
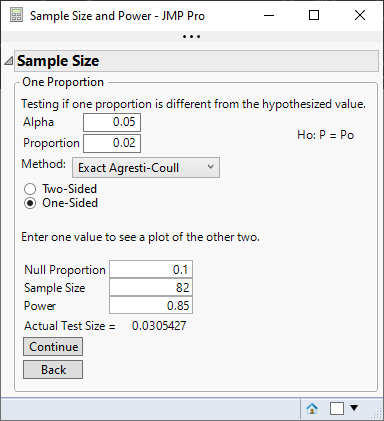
You cannot specify proportion = 0 or proportion = 1, because that hypothesis is untestable. Let's try another realistic scenario. For example, you historically observed failure proportion = 0.1. That proportion is the null hypothesis (nothing changed). You want to test if the change improved (reduced) the proportion failed. You are only interested in an improvement, so use a one-sided test. How much better is the minimum proportion you want to detect? Let's say it is proportion = 0.02. Finally, you might require at least power = 0.85 (85% chance of a significant decision). So you might set up the sample size analysis like this:
Click Continue and the minimum sample size appears.
This example is not a recipe. It is intended to illustrate the thought process and how to use this calculator. Each entry must be decided by you, but hopefully you now understand the meaning and role of each entry.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Sample Size and Power (One Sample Proportion?)
I think that this power calculation is correct for your case. Please see the documentation for help with setting up the power analysis.
You cannot specify proportion = 0 or proportion = 1, because that hypothesis is untestable. Let's try another realistic scenario. For example, you historically observed failure proportion = 0.1. That proportion is the null hypothesis (nothing changed). You want to test if the change improved (reduced) the proportion failed. You are only interested in an improvement, so use a one-sided test. How much better is the minimum proportion you want to detect? Let's say it is proportion = 0.02. Finally, you might require at least power = 0.85 (85% chance of a significant decision). So you might set up the sample size analysis like this:
Click Continue and the minimum sample size appears.
This example is not a recipe. It is intended to illustrate the thought process and how to use this calculator. Each entry must be decided by you, but hopefully you now understand the meaning and role of each entry.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Sample Size and Power (One Sample Proportion?)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Sample Size and Power (One Sample Proportion?)
No, the alternative is that the proportion less than or equal to 0.02, so enter 0.02, not 0.05.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us