R2 (RSquare) and Generallized R2 for the models with nominal response

[#1]

Dear colleagues,

I have got a doubt which R2 is utilized as a metrics for the JMP's models with nominal response, like Partition or XGBoost (available in JMP Pro16). In particular, R2 is used as a metrics for splits counting, which is a key to control the model's effectiveness. For the models with continious response everything is clear for me, but what's the formula and interpretation of R2 for nominal response? 

Regarding Generalized R2, it is not clear yet also - which exactly type of Generalized R2 is used for estimation of model fit: Nagelkerke, McFadden, Craig/Uhler or Cox-Snell? In JMP manual they are reported to be equal, but they are not in fact: here https://statisticalhorizons.com/r2logistic and here: https://stats.idre.ucla.edu/other/mult-pkg/faq/general/faq-what-are-pseudo-r-squareds/

I would highly appreciate your responses and consultation.

With kind regards,

Michael 

[#2]

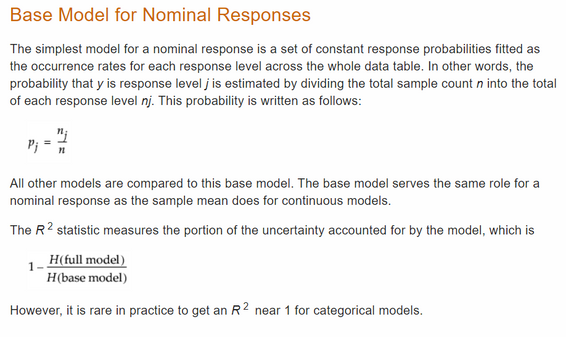
From the JMP help on nominal logistic regression:

This looks like the McFadden type, as your referenced article claims that JMP uses. I did not see anything in the JMP documentation claiming that all of the different RSquare types are the same.

[#3]

Well, my question is composed of two: regarding the R2 for nominal response and Generalized R2. The response about R2 I have just got from you. Many thanks!
As for Generalised R2, the documentation states the identical character of the R2 types. Link + the page attached. So, I ask you kindly to check them and clarify me the case of Generalized R2, if you would not mind.

https://www.jmp.com/support/help/en/15.2/index.shtml#page/jmp/overall-statistics.shtml

[#4 · Accepted solution]

I am highlighting a portion of the JMP help:

Generalized R-Square is also known as the Nagelkerke or Craig and Uhler R2, which is a normalized version of Cox and Snell’s pseudo R2.

This makes it clear that the Generalized R-Square is the Nagelkerke version, which is also called the Craig and Uhler R2. This is consistent with the naming provided on your pseudo-Rsquare article.

The last part of the JMP help sentence says that the Generalized R-square is a NORMALIZED VERSION of Cox and Snell's pseudo R2. This does not mean that they are the SAME. It means that it is the Cox and Snell formula, but is divided by a quantity. See the formulas in your cited article and you will see what I mean.

[#5]

Many thanks for the clarifications! I really got what needed. Have 1 kudo from me to your each answer. Have a nice Sunday!