Prior mean and prior variance matrix in choice design

[#1]

Hi to all,

I am just reading the article Optimal Design of the Choice Experiment - JMP Blog

Here the author writes "When there are three levels in increasing utility order, enter negative, then 0." So far so good. But what if I have e. g. 5 prices like 5, 10, 15, 20, 25 and 30 euros. Which prior means would I take in that case?

30 = -4

25 = -3

20 = -2

15 = -1

10 = 0

5 = 1

And is the prior mean variance still 1 as it is for the factor price in the example?

Many thanks in advance for your help.

Best regards

Herbert

[#2]

The means need to go from negative to positive and sum to zero. So, the above does not work.  I would use something like

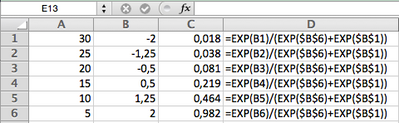
30 = -2

25 = -1.25

20 = -0.5

15 =  0.5

10 = 1.25

5 = 2 <- this one is assumed

The probability that one would choose 5 over 30 is

exp(2)/(exp(2)+exp(-2)) = 0.982

This prior information is already very strong.

[#3]

Hi LTW,

first of all many thanks for your answer! I wanted to follow up with two more questions.

1. "This prior information is already very strong." What would be a reference value for a

• very low
• low
• medium and
• strong

prior information?

2. Why did you choose the range from -2 to 2 and not any other range (e. g. from -4 to 4)?

Best regards,

Herbert

[#4]

Suppose you let your prior means range between -4 and 4. Then, the probability that you would choose the alternative with a prior mean of -4 rather than the one with a prior mean of 4 is

p = exp(-4)/(exp(4)+exp(-4))

/*:

0.000335350130466478

If you really believed one alternative was that much better than the other, then you probably would not need to run an experiment :-)

Typically the fitted coefficients of choice experiments are less than 2 in magnitude. Effects that are much larger than this are so dominant that they swamp the effects of other alternatives.

The idea of supplying prior means is to indicate the relative preference for various levels of each alternative. This allows the design to avoid asking a respondent to choose between a profile with all the good levels versus a profile with all the undesirable levels of the attributes. Such a choice set does not provide any information because every respondent with choose the same profile.

[#5]

Hi LTW,

first of all many thanks for helping such a newbie to statistics as I am! I wanted to to double check with you if I understood the probability function correct. I calculated the probabilities from the example as following. Is this correct?

You write "Typically the fitted coefficients of choice experiments are less than 2 in magnitude." Thats most likely the reason why John Sall uses in his example Optimal Design of the Choice Experiment - JMP Blog a magnitude from 1- till 1. Right?

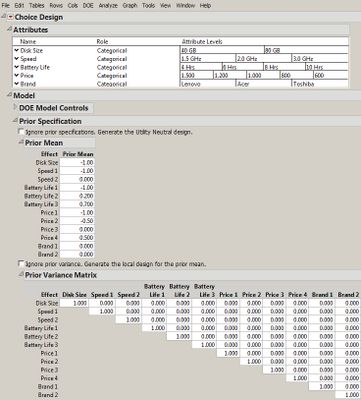
Final question. Based on the example from John Sall I created an slightly extended choice design. Only the attribute brand has no known preferred direction for an level. But the prior variance matrix for brand 1 and brand 2 is still 1.000. Is this correct and do you see any heavy issues in my first choice design?

Many thanks in advance,

Herbert

[#6]

Hello,

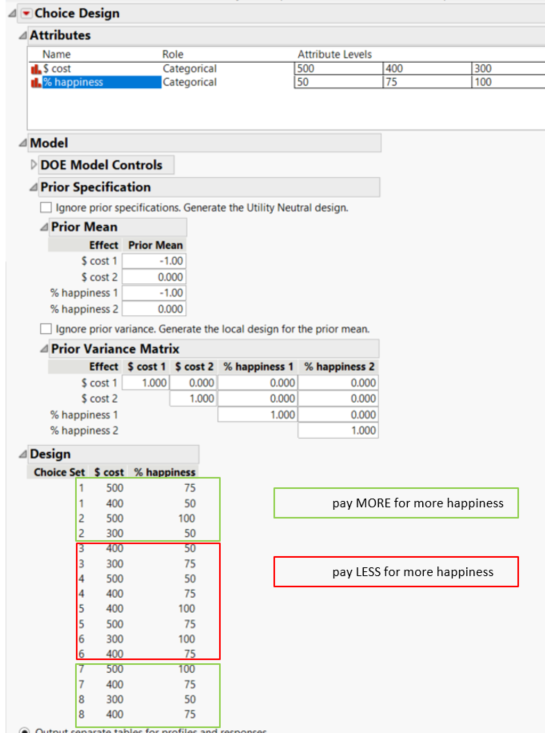
I thought that I understood prior mean based on the above and some other posts/videos, but I am not understanding why the choice design, attached, is being generated. The attributes cost and happiness are listed in increasing order of value, and the prior mean is set as shown in the computer example above. This should prevent choice sets being displayed that offer the best of both. However, as shown, sets 1,2,7,8 are correct (more happiness costs more), but the others give the option of selecting more happiness for a lower cost. 

Besides the issue of happiness should not have a cost, what am I missing here? All of the choice sets should make you choose whether you want to pay more for happiness. 

Thanks in advance.