- JMP User Community
- :
- Discussions
- :
- Is there an easy way to get the FWHM (full width half max) of a curve using JMP?
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Is there an easy way to get the FWHM (full width half max) of a curve using JMP?
Dear JMP community,
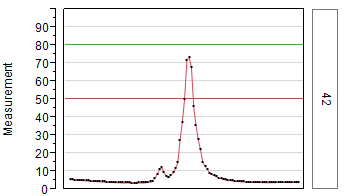
Is there an easy way to get the FWHM (full width half max) of a spectral curve using JMP?
Finding the minima and maxima are fairly straightforward, but then calculating the FWHM seems to be much more laborious in JMP, especially if the data is sparse and we would like to interpolate to get more accurate intersections between the peak and the FWHM value.
In my case, the peak is often assymetric and can have several side peaks and both global and local minima.
Here is the basic approach that I would take for finding the FWHM:
- Use Summary statistics to find the max value over the desired range.
- Then Join the new and old data tables to get the matching peak wavelength where each maxima occurred.
- One could also do this for the minima to the right and left of the peak and then find the average value for the half-max (i.e., with the baseline subtracted, using the average value of the two adjacent minima as the baseline).
- Use the Summary Statistic approach, find the values that best-match the half-max (just below and just above the half-max) on either side of the peak wavelength (short wavelength and long wavelength side).
- Join the new and old data tables to find the wavelengths where these best-matched values occurred.
- May want to interpolate to get a more accurate value for the two intersection points of the spectra with the half-max value.
- Then calculate the FWHM and spit-out the relevant data points.
If you know of any other approaches, please let me know. Perhaps I could calculate the 2nd derivatives and use the inflection points on either side of the peak to get an alternate measurement of peak width. Are there approaches for taking derivatives in JMP?
- © 2024 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us