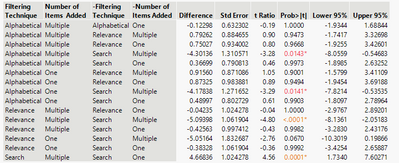
The context of my data is from an e-commerce website. I am measuring the number of errors made based on different independent variables: Filtering technique (Alphabetical, Relevance and Search) and Number of Items added (Add one at a time, or add multiple items to cart at once). As the number of errors made is 0 most of the time, I have opted to use the Zero Inflated Poisson Regression. When I performed the pairwise Tukey HSD comparison, I get the following picture.
How can I interpret the t ratio and p values in this case? For example, for Filtering Technique = Alphabetical and Number of Items added = Multiple, vs Filtering Technique = Search and Number of Items Added = Multiple, t ratio is -3.28 and p=0.0143. Does this mean that Search,Multiple number of items added has a higher significance of errors than Alphabetical,Multiple number of items added (since t is negative)? This seems to contradict the data I have, where there there is no errors made in the scenario where filtering technique is search and there are multiple number of modules added
turkey hsd pairwise tests
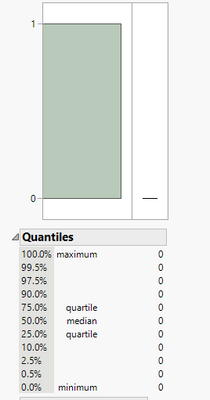
Distribution of data (Alphabetical, Multiple)
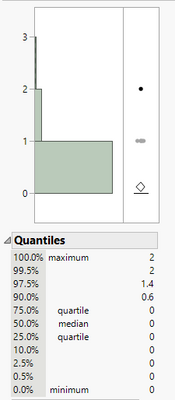
Distribution of Data (Search, Multiple). Note there are 0 errors made