How are Gamma-Poisson Distribution Fit Parameters Determined? They differ from a least-squares solution.

[#1]

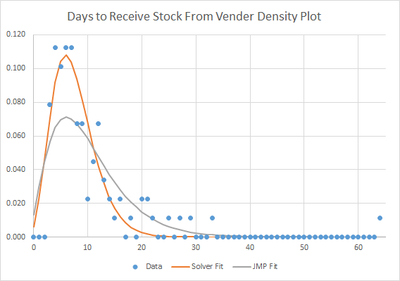
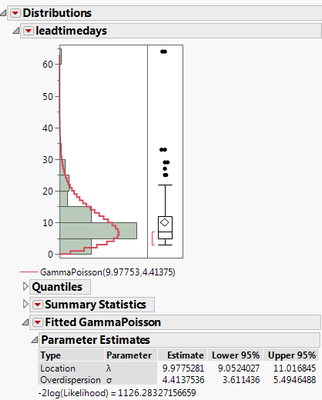
The distribution platform estimates lambda and sigma parameters for a Gamma-Poisson distribution of the attached data to be 10.0 and 4.4.  A separate least-squares method finds 7.5 and 2.1.  I am not proposing the orange line to be better than the gray (JMP) only that it fits my current needs better.  For other data sets the parameters found by both systems are fairly close.

Does anyone have an idea as to what is happening here?

Sum of error squared

JMP: 0.014

Solver: 0.0086

Thanks,

Isaac

[#2 · Accepted solution]

normaly with MLE (maximum likelihood estimation)

https://en.wikipedia.org/wiki/Maximum_likelihood_estimation

the -2log(Likelihood) in your picture is a good hint that it's probably used.

[#3]

Thank you maurogerber.

I figured this out shortly after posting the question but then couldn't edit the question as the site was down. :-(