- JMP User Community
- :
- Discussions
- :
- DoE with one factor constrained to be in a ratio with another factor
- JMP 19 is here! See the new features at jmp.com/new.
- Register to attend Discovery Summit 2025 Online: Early Users Edition, Sept. 24-25.
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
DoE with one factor constrained to be in a ratio with another factor
Is there a way to use the Custom Design to generate a DoE where one factor is set to a ratio of another? For example, if I have factors A, B and C, each at two levels, however the two levels of C are 1:1 with B or 5:1 with B. This obviously creates more than 2 real levels for C and some of the combinations are not allowed because they don't maintain the ratio. Treating the ratio (i.e. 1 and 5) as the "levels" seems wrong. I know this violates basic assumptions.
I tried using the disallowed combinations filter but I'm not having success. Any advice would be appreciated.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
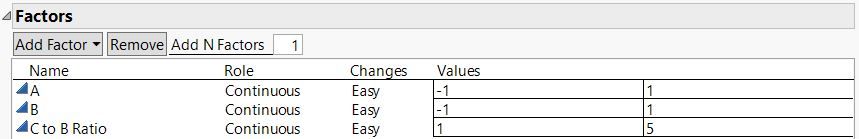
Correct me if I misunderstood your situation or question. I have three continuous factors, A, B, and C. I want to design an experiment, though, for a model for A, B, and the C to B ratio. Here are the factor definitions:
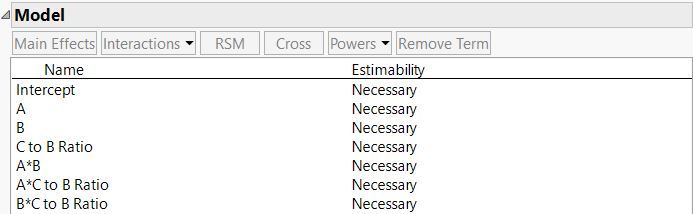
I can choose any linear model, in this case I included interaction terms:
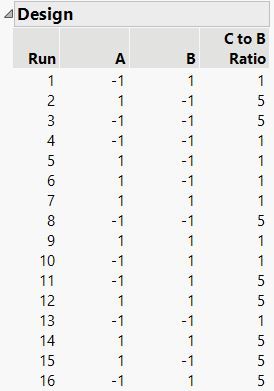
I ask JMP for 16 runs:
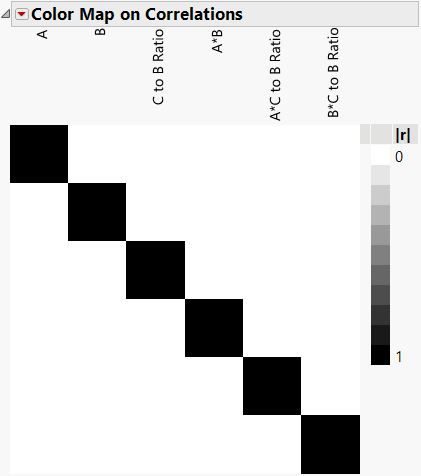
The color map on correlations shows that these estimates are orthogonal:
The lack of correlation is a matter of the model and the number of runs, but I can achieve a balanced design in this case to demonstrate that orthogonality can be achieved. Another column must be added to the data table to determine the factor levels for C in each run.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
I don't know the specific situation so I can't be sure of what are the practical, engineering or statistical issues, but here are my thoughts:
1. If you are not concerned with the interaction of C with B, you could nest C in B. In this case you would have 2 different levels of C for each level of B.
2. If you already know the dependence of B & C, you could just create a variable with is at 2 levels and those levels include the combinations of B & C that are appropriate. Example, in paper manufacturing determining what factors affect paper weight, I could have 2 factors Virgin Pulp & Reground Pulp. I could experiment on each of those as separate factors or as 1 factor with 2 different ratios.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
I am not sure that there is anything wrong with using factor C as the ratio (multiplicand) for B. You are building an empirical model, so as long as you think of C as the ratio, and use it as such in model predictions, it should be OK. Here is an example:
You can add a data column after you make the data table to determine the actual level of C to maintain the ratio.
You use C in the model and Actual C in setting up each run. The model could be used to find the optimal A, B, and ratio (C).
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
@Mark_Bailey Mark thanks for this reply. So, as long as I restrict all inference, assessment of residuals, and predictions etc. to the ratio, this will not violate any assumptions of the model? If the design is orthogonal does it remain so?
Still have many questions but I just want to be sure that this practice is legitimate. Thanks!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
I do not understand what you ask when you say, "If the design is orthogonal does it remain so?" To me, that question is like asking, "If the car is red, does it remain red?" so I think I missed something.
This situation reminds me of many cases with a categorical factor. The levels are just surrogates for one or more continuous factors that remain unidentified. But the model with the discrete levels is still valid and useful.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
@Mark_Bailey what I meant by the orthogonality question was, if the underlying factor doesn't vary independently of another factor, we can still treat the ratio as independent.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: DoE with one factor constrained to be in a ratio with another factor
Correct me if I misunderstood your situation or question. I have three continuous factors, A, B, and C. I want to design an experiment, though, for a model for A, B, and the C to B ratio. Here are the factor definitions:
I can choose any linear model, in this case I included interaction terms:
I ask JMP for 16 runs:
The color map on correlations shows that these estimates are orthogonal:
The lack of correlation is a matter of the model and the number of runs, but I can achieve a balanced design in this case to demonstrate that orthogonality can be achieved. Another column must be added to the data table to determine the factor levels for C in each run.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us