Confidence Intervall DOE

[#1]

Hello there,

I have some issues concerning the evaluation of my results of a Design of Experiment which I conducted.

To Confirm my Response-surface-model, I conducted 6 confirmation runs. To check whether my model is able to describe the system I need it's confidence interval. But there is a problem. First I used the profiler in the prediction-model-window. But those values seemed to be to small, so I went on "Save Columns" and I saved the Formulas for "Individuals Confidence Formulas".

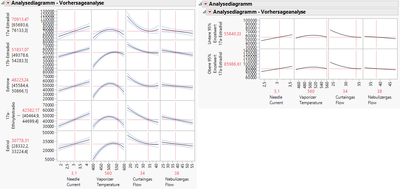
Using them in the prediction profiler graph, the results were different from those in the fit model window (See picture below) . How is that possible?

My second question is, what is the difference between the two formulas "Mean Confidence Limit Formula" und "Individual Confidence Limit Formula"?

I hope anyone can help.

Alexander

(I'm not a native speaker so I hope you can understand my problem even if there are some issues concerning language)

[#2]

I forget to say that on the left picture you see 5 responses, the first response is the one you also see on the right picture. Why are the Limits for 95%-Confidence-Intervall different?

[#3]

The confidence interval is for the mean response. The prediction or individual interval is wider because covers both the uncertainty in the mean and the error variance of the individual observations.

Alternatively, you could use a one-sample t-test with your sample of 6 observations against your predicted or expected mean.

[#4]

Thank you for answering my question.

If i would use a t-test to compare my experimental results with my predicted value I would calculate my t-value using the standard deviation of my repeated measurements?

If I want to compare my experimental results (every confirmation experiment was measured three times to calculate the mean) with my predicted values is it better to chose the "confidence interval for the mean" or the "confidence interval of the individual measurements"?