- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Discussions
Solve problems, and share tips and tricks with other JMP users.- JMP User Community
- :
- Discussions
- :
- Computing statistical tolerance intervals in JMP
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Computing statistical tolerance intervals in JMP
Hi,
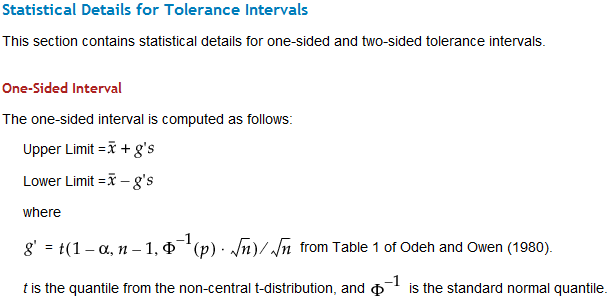
I am looking into the calculation of one-sided tolerance intervals. Searching the documentation, I found the following paragraph:
Now, please correct me if I am wrong, but as I understand it:
- s is the standard deviation of the data samples
- is the sample mean
- n is the number of samples
- 1-α is the confidence
- t is the noncentral t inverse cumulative distribution function
- and finally is the inverse cumulative normal distribution
The reason I am asking is that I am having difficulties recreating the tolerance interval calculated by this method in matlab. Please let me know if there is something here that I got wtong. Thank you.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Computing statistical tolerance intervals in JMP
It looks like you have it correct; for the one-sided case, g' can be calculated exactly (as shown in the documentation). For the two sided case, however, a variety of approximations are available and some are better than others. But, be warned, it is not the case that two one-sided tolerance intervals (lower and upper, respectively, each at 1-alpha/2 confidence) gives the same interval as a two-sided tolerance interval at 1-alpha confidence.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Computing statistical tolerance intervals in JMP
It looks like you have it correct; for the one-sided case, g' can be calculated exactly (as shown in the documentation). For the two sided case, however, a variety of approximations are available and some are better than others. But, be warned, it is not the case that two one-sided tolerance intervals (lower and upper, respectively, each at 1-alpha/2 confidence) gives the same interval as a two-sided tolerance interval at 1-alpha confidence.
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us