- JMP User Community
- :
- Discussions
- :
- Re: Can Polynomial fit preference be set for not centered?
- JMP 19 is here! See the new features at jmp.com/new.
- Register to attend Discovery Summit 2025 Online: Early Users Edition, Sept. 24-25.
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Can Polynomial fit preference be set for not centered?
Hi,
The standard polynomial fit returns a centered set of fitted parameters. I get from a statisticians perspective why this is done, though in most of science and engineering this is not the default way of looking at equations. I know I can work around this by going to fit special selection, and click, click, click, click...
Is there a way to set the default behavior of polynomial fitting to use non-centered parameters? I have looked an preferences but nothing is jumping (small pun) at me.
Andy
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
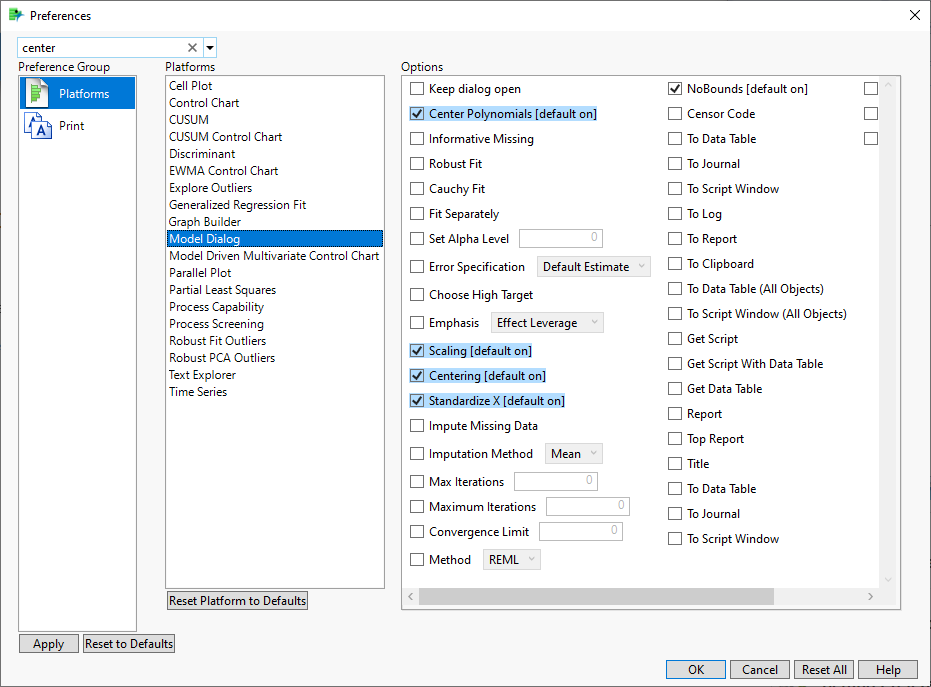
I found this preference:
Regarding acceptance and success of DOE in your field, you might look up work in the past decade by Chris Nachtsheim about the use of Dimensional Analysis with DOE. That marriage might get more attention in your area.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
I'm a little confused by this statement: "though in most of science and engineering this is not the default way of looking at equations." As an engineer when developing a least squares model, I start with the Y intercept and then understand how much each variable in the equation "moves" the response. What do you mean by this is not how most scientists and engineers look at equations? I'm asking to understand your point of view. You can, during fit model, select the no intercept option.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
First of all, you are free to specify practically any linear or non-linear model you want for your data. Sometimes there is a theoretical basis for the model. Often, there is not. As such, empirical models often do not have a completely physical interpretation like their theoretical counterparts. That is to say, each term or parameter has a role, but not necessarily represent a direct physical attribute.
The constant term in a polynomial expansion that is used as the linear predictor in regression is an empirical model. The intercept could be interpreted as the y-intercept at x=0, but that is not its only purpose or use. It is the mean response at the origin, which is useful if your predictors are centered. Centering is not based on a theoretical model. It is based on the performance of the regression method. It improves the power of the hypothesis tests of the parameters by reducing the correlation of the parameter estimates when terms above the first order are included for example.
Finally, there might be other contributions to the response y even when the predictors are all at zero leading to a non-zero intercept.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
First, the request again: Is it possible to set preferences for polynomial fits to be not-centered. I know I can go through workarounds, but I would like to improve my work flow.
The more philosophical point: My domain area is material science specifically process and structures for semiconductor solar, battery and I am supporting R&D teams. One observation I have from engineers (over 35+ years and many companies and teams) is they hate DOE mostly because it is empirical information. My favorite story: CEO would give $100 bonus for every DOE run and CTO would fire anyone suggesting running a DOE and it was the same company. The definition of mixed messages. For DOEs, if the solution falls within the test space great much more often than not is does not. So they need to make changes and an empirical model is of limited value to them because they need to tie the results back to theory (or theory of operation for engineering projects). For this reason engineers and scientists are more comfortable with single variable experiments specifically because they need to make the connection.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
I found this preference:
Regarding acceptance and success of DOE in your field, you might look up work in the past decade by Chris Nachtsheim about the use of Dimensional Analysis with DOE. That marriage might get more attention in your area.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
Thank you Mark,
Given the extremely large range of preference settings, if would have taken me quite a while to find it.
Andy
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Get Direct Link
- Report Inappropriate Content
Re: Can Polynomial fit preference be set for not centered?
Notice the Filter box in the upper left corner? It's your friend. Good to remember in the future!
Recommended Articles
- © 2025 JMP Statistical Discovery LLC. All Rights Reserved.
- Terms of Use
- Privacy Statement
- Contact Us