Yates' order in JMP

[#1]

Hi!

Minitab numbers the runs in Yates' order (standard order in Minitab).

Is there a way to sort the runs in Yates' order in JMP? 

I want to create Yates' order for 18 factor design but Minitab is limited to 15 factors.

Thank you!

[#2 · Accepted solution]

One of the choices before you click Make Table is the Run Order. This option defaults to randomization. Click on the drop-down menu and select Keep The Same, Sort Left to Right, or Sort Right to Left. One of these (I believe) is Yate's order.

Why do you want to sort the runs this way?

[#3]

@Mark_Bailey, thank you very much! It helped.
"Sort Right to Left" is indeed a Yates' order.

[#4]

Sorry, it worked for 3 factor full factorial but it none of the options woked for 15 factor 2-level fractional factorial.

They are different from Minitab's standard order.

[#5]

You can use the Change Generating Rules section after you select a design (e.g., 32 runs, unblocked, regular fractional factorial) to obtain a different fraction. It will not necessarily have the same resolution as the original fraction that you selected and will have different aliases.

JMP produces a minimum aberration design for the simplest alias structure. There are many other fractions (e.g., 2^(15-9)) possible, of course, each with different aliases. JMP arbitrarily chooses the first n columns for which the design would be a full factorial (e.g.,2^6 = 32, so  n = 6) and then introduces the remaining factors(e.g., 9) by taking higher-order columns from the model matrix that is used in the regression analysis to estimate the parameters. These columns are no longer available for estimation.

Generally speaking the generating rules and the resulting fraction with its associated aliases are not that important because we do not have prior knowledge sufficient to know which factors and factor effects are important to choose one fraction over another. That is, you could permute/exchange the factors and still expect the same performance from the design. We depend in the key screening principles for any of the factions to succeed.

Why do you need a particular fraction?

[#6]

Thank you for your help!

I need Yates' Order for simulation purpose. I am trying to kind of reverse engineer DoE.

I simulate responses from predefined estimates. Since I know (1) from Yates' order, effects A, B, C, etc.

Then I can calculate simulated responses using Simultaneous Equations. 

I have successfully done this for full factorial. Now I want to do this for fractional factorial.

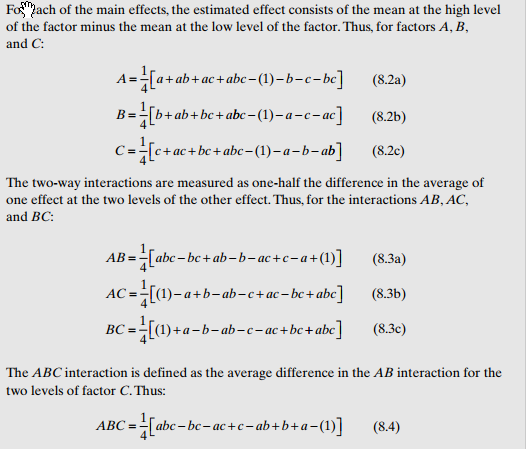
I use these equations:

[#7]

You might want to consider vectorizing your computations. The matrix form of the calculations would not be affected by permutations of the rows. This way is the same as the simultaneous equations but more efficient and flexible. You have Y = X*B for the simultaneous equations.You start with the design matrix D (one of your factorial designs) and expand it into the model matrix X for estimation. The X matrix contains columns for each effect (intercept (1), main (X1), interaction (X1*X2), quadratic (X1*X1), and so on). The columns in X will be used to estimate the effects A, B, C, AB, AC, and so on instead of your equations. The B column matrix contains the parameters. Now B = Inverse( X` * X ) * X` * Y. The row order of the runs does not affect the result B. Their order is determined by the order of the columns in X, which you control.

By the way, the form of the calculations that you are using only works with factorial designs. The calculations depend on the balanced nature of the design to work. These early designs were possible to make and analyze by hand, a great benefit in the 1920s. This fact, however, led to a belief that designs must be balanced that lingered until recently, which is not true. Using regression instead of the simultaneous equations can properly compute the effect of any design, even if it is not a full factorial or regular fractional factorial design. I prefer the general way of estimating the effects that handles data from any design.

[#8]

@markbailey, that’s great, thank very much for this suggestion!

Now I understand that Yates' standard order wouldn't be appropriate for fractional-factorial design.

Could you please specify the name of the method that you described or some keywords, so that I could use them to search additional information about the method in google books? I just want to know how exactly can I execute these calculations in R or JMP or Excel. I used R scrip in order to solve simultaneous equations.

I heard about the method "Yates' inverse".

And here is another technique described by Paul Berger in his book:

[#9]

The method is linear regression.You can use JMP Analyze > Fit Model to specify the the response column and the terms in the linear predictor and then click Run to estimate the parameters for the desired effects.

Alternatively, you can send the Get As Matrix message to the data table with your design and then use the matrix language in the JMP scripting language to compute the estimates yourself.

I don't know your background but this problem is pretty standard for linear regression.

[#10]

I am from psychology field. Yes, I know about Basic Statistics and Linear Regression, but my purpose was to derive responses for each row from effects and (1), not vice versa.

Or do you mean that I can calculate responses for each row (treatment combination) from predefined effects (A, B, C, (1)) using linear regression? Unfortunately, I could not understand how.

What I did for full-factorial:

1. Created Yates' Order in a design table

2. Created equations where values of (1), A, B, C, AB, BC, AC, ABC where predefined and a ab ac abc b c bc were unknown.

3. Solved simultaneous equations in order to find values of responses for each rows that is a ab ac abc b c bc.

Because I took the example where responses were already known I could compare the results and they were correct.

I thought I could use the same process for deriving responses for fractional-factorial.