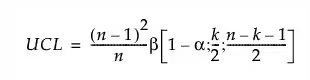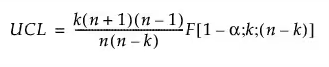Help understanding T^2 UCL calculation in MDMVCC (automatic vs manual calculation)

[#1]

Hi All,

  I have been working with the Model Driven Multivariate Control Charts for a little bit now and was just refreshing my memory/skills on the subject when I started to delve deeper into some of the statistical details for the platform. In the process, I thought I'd try to do a manual calculation for the T^2 upper control limit, according to how it's defined in the JMP online help, here; note that there are two definitions for the UCL, historical and current data.

  Both are similar, and other than the Beta or F distribution, only depend on the number of rows, n, the number of principal components, k, and 1-alpha. By default, alpha is 0.05, so 1-alpha = 0.95.

  As an example, I'm using the Steam Turbine Historical.jmp file -- in fact, you can find the same example for this in the online help, here. When you run the MDMVCC platform and select all the columns (in this case there is no historical row data, you use all the rows), the T^2 plot suggests an UCL of about 7. When I try to calculate the UCL manually using a column formula, I get very different numbers. For the Beta distribution version, I get 26.04 and for he F distribution version, I get 1.91.

  I'm guessing the reason is because of how the distributions in the UCL are defined/referenced in the JMP help is somehow different than how the functions are called/used in a column formula, or in JSL code. Below is how I am defining the column formulas. Compare to the above definitions.

Beta

F

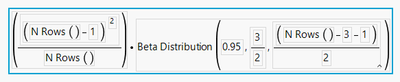
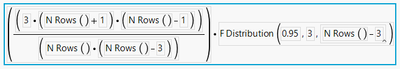
  According to the Scripting Index, the arguments to these functions sound a little different than the definitions given in the online help. I've even tried swapping the last two arguments in the distribution functions, but the UCL still doesn't match up.

  Not surprisingly, running a PCA analysis with 3 components results in the same Median and UCL as that shown for the MDMVCC platform. Same with DModX. Hopefully someone can help explain the discrepancy I'm getting and why. I'd like to be able to reconcile the difference in the UCL values I get manually vs those the MDMVCC and PCA platforms generate.

Thanks!,

DS

[#2 · Accepted solution]

Hello @SDF1 ,

It seems that you need to use the Beta Quantile function instead of the Beta Distribution function.
It is the same for F Distribution function. You need to use F Quantile function.
Hope it helps.

Yuichi

[#3]

Hi @yuichi_katsumur ,

  Thanks for the clear explanation. It wasn't obvious from the statistical details in the online help that one needed to use the quantile function of the distribution in question. But, now that you've cleared this up, I can verify that it works in both cases to calculate it manually, whether for historical only data or historical plus new data. Thanks again for clarifying my mistake!

Thanks!,

DS